/615412b8-092e-48dd-926a-3ac4971dc7cf.png)
Right Triangle Pythagorean Theorem
Review Rating Score
The Pythagorean Theorem is a fundamental concept in geometry that describes the relationship between the three sides of a right triangle. Whether you're a student struggling to understand the theorem or a professional looking for a convenient tool to use in your work, BizzLibrary.com has got you covered.
What is the Pythagorean Theorem?
The Pythagorean Theorem states that the square of the hypotenuse (the longest side) of a right triangle is equal to the sum of the squares of the other two sides. This theorem can be represented by the formula a² + b² = c², where a and b are the lengths of the legs of the triangle and c is the length of the hypotenuse.
How to Use the Pythagorean Theorem?
The Pythagorean Theorem can be used to solve a variety of problems in geometry. One common application is to find the length of one side of a right triangle when the lengths of the other two are known. For example, if you know that one side is 3 units long and the other is 4 units long, you can use the Pythagorean Theorem to find the length of the hypotenuse:
a² + b² = c²
3² + 4² = c²
9 + 16 = c²
25 = c²
c = 5
The length of the hypotenuse is 5 units.
Using the Pythagorean Theorem to Find the Nearest Point
Another application of the Pythagorean Theorem is to find the nearest point to a given point on a line. This can be useful in a range of contexts, from city planning to game development. To find the nearest point, you'll need to use the Pythagorean Theorem to measure the distance between two points in space.
For example, let's say you want to find the nearest point on a line to a given point (4, 2). First, you'll need to find an equation that describes the line. Let's say the line is described by the equation y = 2x - 1. Then, you'll need to find a point on the line that is closest to (4, 2). To do this, you'll need to:
- Find the slope of the line: The slope of the line is 2. This means that for every one unit you move to the right, you move up two units.
- Find the coordinates of a point on the line: You can pick any value of x and plug it into the equation to find the corresponding value of y. Let's say you choose x = 2. Then, y = 2(2) - 1 = 3. So, the point (2, 3) is on the line.
- Use the Pythagorean Theorem: Calculate the distance between (4, 2) and (2, 3) using the Pythagorean Theorem. This gives you:
√[(4 - 2)² + (2 - 3)²]
√(2² + (-1)²)
√(4 + 1)
√5 ≈ 2.236
The nearest point on the line to (4, 2) is (2, 3), and the distance between them is approximately 2.236 units.
Download Our Pythagorean Theorem Tool Today
If you're looking for an easy-to-use and comprehensive tool for understanding and applying the Pythagorean Theorem, look no further than BizzLibrary.com. Our Pythagorean Theorem tool is available for download in DOCX format, making it easy to incorporate into your work. Whether you're a student, teacher, or professional, our tool has everything you need to master this essential concept in geometry.
Is the template content above helpful?
Thanks for letting us know!
Reviews
Chi Curtis(6/14/2023) - NZL
Perfect!!
Author. Content was provided by:
Elizabeth Davis
Elizabeth is from the sunny desert city of Phoenix, Arizona. She is thrilled to connect with professionals and like-minded individuals who share a passion for social technologies, content creation, and the exciting possibilities that AI brings to the world of social media. Her hobbies are hiking, climbing, and horse riding. Elizabeth has a master's degree in Social Technologies that she received at the ASU (Arizona State University). As a freelancer, she mostly contributes content related to IT. This includes articles on templates and forms provided by our community.
Follow Elizabeth
Last modified
Our Latest Blog
- The Importance of Vehicle Inspections in Rent-to-Own Car Agreements
- Setting Up Your E-mail Marketing for Your Business: The Blueprint to Skyrocketing Engagement and Sales
- The Power of Document Templates: Enhancing Efficiency and Streamlining Workflows
- Writing a Great Resume: Tips from a Professional Resume Writer
Template Tags
Need help?
We are standing by to assist you. Please keep in mind we are not licensed attorneys and cannot address any legal related questions.
-
Chat
Online - Email
Send a message
You May Also Like

Sample Leadership Retreat
Seedling Price List - Affordable Saplings for Your Garden | Buy Best Quality Seedlings Online

Colored College Ruled Paper
College Ruled A Size Paper Template
Get Fit as a Family with our Printable Workout Log | Track your progress and stay motivated

Download Beautiful Birth Announcement Template

Fitness Therapy Schedule Template - Water, Exercise Classes, Stretch
Excellence in Education

Download MLA Reference Style Format: The Ultimate Guide with Examples

Sample Article Summary Template - Write Effective Summaries Easily
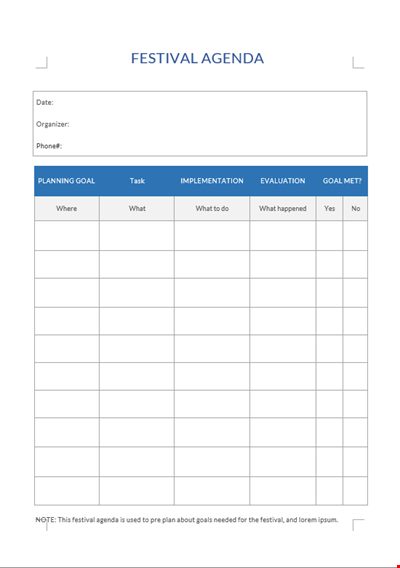
Festival Agenda A4

Fact Sheet Template - Health, Water, Copper, Drinking Levels

Social Event Program Layout
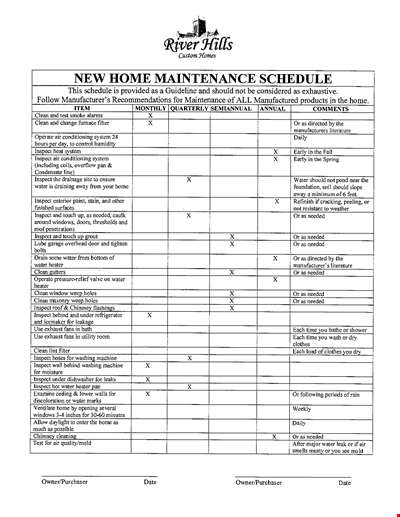
Organize Your Home Maintenance with our New Template

Church Leadership Qualities Example

Non Profit Board Agenda